The puzzle looks like this:
And here is what happened.
The first 10 minutes were spent coming to terms with the problem - what would a quarter look like?
Well we found lots of things that it wasn't. We soon realised that the cross could be made of five squares, so colouring in one or two of these was not going to make a quarter.
Back to the drawing board.
This was one of my favourite solutions because it showed me the struggle that was going on. It was like a window into the mind of the student.
"So, what if I do this? Hmm, no I don't think so. What about... yes that might work!"
It was the process of deciding on a "proof" - how to show I had found a quarter. Along the way, I am going to need to eliminate a few ideas but this is all part of the learning process.
While doing this task, I pulled the class back in for a "chat" about 3 times. This helped to clarify the question and also to discuss any important information we had discovered. This was shared with the class to help progress the solutions. We also had a break of a few hours and revisited the task in the afternoon.
And here are a few of the ideas that emerged:
1. You can use one of the "legs" of the cross and add it to 1/4 piece of the inside square.
What the students had seen was that 1/5 + 1/20 = 1/4 although they were not able to articulate this.
2. Using a line that passes through the centre of the cross
This was a good idea. If you extrapolate from this you will find that this method produces an infinite series of solutions as you rotate around the centre point. We only represented 3 solutions.
3. There is a left handed and a right handed house
Interesting - same idea but reflected.
4. Colour makes everything look good
So Amie - hope you like our pics. I think they look great. The kids had a really good time and it was difficult but we got some good learning out of it.
Now, I wonder what other shapes we could divide up...





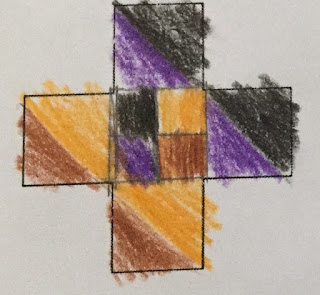





No comments:
Post a Comment
Any comments you would like to make?