miniMaths Book 2

The Background

Following our successful application in 2018 for an ACT Government Nature Play grant, I submitted an application on behalf of the Canberra Maths Association for another grant in 2019. As we had planned in 2018, we again proposed to:

- write 10 inquiries/tasks/lessons/resources to be used outside in the natural environment
- run a series of workshops with preschools to share these resources

I am very pleased to announce that we were again successful in our application and got the grant. A group of about 10 interested preschool teachers met together one afternoon earlier in the year at Radford College, my school, and brainstormed some ideas. It was a productive time. I went away with pages of notes that I wrote up as the miniMaths Book 2. 

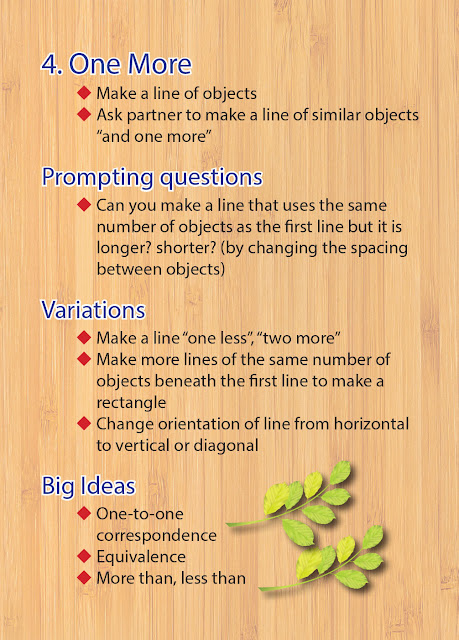
The 10 tasks we came up with were:

1. Which hand? - an exploration into probability
2. Hands and feet - measuring with informal units
3. Symmetrical mandala - building a symmetrical pattern
4. Leftovers - what to do with remainders when dividing
5. There and back - giving directions to describe a journey
6. Pattern dance - using symbols to represent the parts of a dance
7. Flower count - collecting data about flower colours and representing the data as a "graph"
8. Shape detective - finding shapes in nature
9. Treasure sort - sorting objects based on shared and unique features
10. Fill the pot - how many cups of sand does it take to fill a saucepan?

A beautiful flower mandala created by the wonderful students 

at Orana Steiner School

The 10 tasks make up Book 2 of the miniMaths program. Every preschool and early learning centre in the Australian Capital Territory will receive copies of the book early in 2020.

The 10 tasks have also been uploaded as a website that is available to anyone, not just ACT teachers:

http://www.minimaths.com.au 

Over the next week or so, I will post a bit more detail about each of the 10 tasks. Maybe you will find them useful. I would be keen to hear any feedback.

And any Canberra teachers - keep an eye out for the miniMaths workshops rolling out over January and February.

Regards.

1 10 100 1000 10000 100000 1000000

Let's have a look at place value with Year 4.

The Australian Curriculum says:

Recognise, represent and order numbers to at least tens of thousands (ACMNA072)

So we did.

I got some 1mm grid paper and we started off with one tiny square.

Then we cut out a row of 10.

Then we cut out a square of 100.

Then a row of 1000.

It started getting a bit technical here. It seemed like I had hit a developmental barrier - a place that required some additional cognitive effort. I was a bit surprised but we worked our way through. 

Obviously (to me at least) 10 000 was going to be 10 rows of 1000. It didn't appear to be that obvious to the students. Why was there this hesitation? Perhaps this is why the Australian Curriculum identifies this as the target size to go for in Year 4. However, once we started counting by groups of 1000, we soon got the idea of what 10 000 looked like.

One of my ambitions with this task was to get a visual experience of what happens when we start multiplying by powers of 10. Also to get the connection with the decimal place value system we use - more of this later.

Once we had 10 000 sorted, it was a smaller step for mankind to decide what needed to be done to represent 

100 000. It's 10 of the 10 000 squares. 

At this point, a few students began to see a pattern forming in the way we were representing the numbers:

1 = square

10 = line

100 = square

1000 = line

10 000 = square

100 000 = line

1 000 000 = square...

Well, we haven't quite finished the 1 000 000 square but we are nearly there.

And the classroom is a bit of a mess - just the time when a member of the executive chooses to walk in...

This is the room after we did a bit of a tidy up.

So now we have models of 1, 10, 100, 1000 etc.

A valuable experience.

But the other connection I wanted to make was with the decimal place value system. Because with my models, I can now choose any of the samples to be the unit - it doesn't have to be the tiny little square = 1. It could be any of the other models we have made. The very biggest square, that we thought was 1 million when we made it, may in fact become 1 unit, or 1 thousand or 1 anything. 

And if that is the case, then what is the value of the other numbers? And where does that decimal point need to go?

Time to start playing...

miniMATHS - 7. Make a Star

This is my favourite.

I know - you're not meant to have favourite children.

But this one is where it all started. This was my first idea for the miniMaths series of tasks. So I think I had given it more thought and probably used it as the benchmark for what I imagined the other tasks would look like.

And this one has real maths behind it. As you build up your star, you are adding a constant value to your pattern - one rock for each arm of the pattern. It is symmetry. It is an arithmetic sequence. It is pre-algebra. It is the connection between repeated addition and multiplication. And it is fun. It makes a cool looking pattern.

This task is linked to EYLF - Outcome 4, focusing on the disposition of the learner. The task itself is open enough to allow creativity and curiosity. It can be done as a collaborative and cooperative task. It can help students develop confidence and perseverance. 

Give it a go - it is a great task.

miniMATHS - 6. Stacking

This is fun. I know - I could spend hours doing it. In fact, I used to get kids to do it when I was on playground duty and I would challenge them to get 5 rocks stacked on top of each other.

But where is the maths?

Well, there are a couple of ideas here that need to be explored.

1. Have you ever worked with kids (probably Year 2 or Year 3) who had the idea that when constructing a "sum" (or algorithm - addition or otherwise) you had to start with the big number and then perform the subsequent operation on it? And isn't there an addition strategy for counting on that says you need to start with the big number first? Is this somehow related to stacking up rocks or other items where it is a good idea to put the big one on the bottom of the stack? Please note, I'm just asking the questions here - not providing the simple answers.

2. Or could this be a revelation of the mathematical concept of combining different values to create new one?

3. Or is it an exercise in balance, similar to an equation where one side has to equal the other?

4. Or are we playing the mathematical idea of prediction - what happens if...?

Perhaps the EYLF will give us some insight:

The EYLF certainly talks about "balance" as an important element of growth. This task provides a neat entry point for this conversation.

This task will also provide an opportunity for students to develop perseverance - to try to get that stack higher and higher. It will also encourage students to reflect on their unsuccessful strategies and modify and improve on the performance. 

So, just as in the "Shadows" task, this activity may not appear at first glance to be explicitly "mathematical", there is great capacity for students to explore deep mathematical thinking by attempting the stacking task.

If you haven't seen it yet, there is a miniMaths website:

http://www.minimaths.com.au

And for people based in the Canberra region, I am running some workshops in preschools and early learning centres over the next six weeks. Details can be found on the miniMaths website.

See you there. 

miniMATHS - 5. Shadows

Sorry about the delay of a few days there - we went back to school last week and I have been a bit distracted getting the class into shape..

So here we have possibly the first major deviation in the miniMATHS resource tasks. This task, "Shadows", moves away from activities that have direct mathematical concepts as a focus, such as extending patterns or investigating area, to a place where we are investigating a natural phenomenon, such as shadows, using mathematical skills and knowledge. No longer playing directly with maths - now we are using maths as we play with something bigger.

Shadows are fascinating. There is so much that we can learn by observing them. They are a great leaping off point for exploring time; they are an explicit example of change; we all have that follows us around - and there are lots of games you can play with them.

All of the things that are suggested as activities associated with this task can be investigated using mathematical language. This is a key opportunity to develop our use and understanding of words that describe position, shape, movement and change.

This task is a great way to engage directly with the natural environment in an explicitly mathematical way. We can record changes over time using the outlines of shadows drawn on the ground. We can discuss the position of the light source relative to the object casting the shadow and the shadow itself. 

The EYLF talks about knowledge of and respect for the natural environment. This task will give students a greater understanding and appreciation of what happens in the world in which they live, helping them to be connected with it and to learn respect for it.

Is it "maths by stealth"? Sneaking a bit of maths into a fun exploration of a cool phenomenon? 

Well, I would say no - this is what real maths is: using our skills and knowledge to make sense of the world around us. And hopefully we can start this in early year education with young kids, engaging them as mathematicians to learn more about their world.

miniMATHS - 4. One More

Task 4 - One More.

Seems like a very simple task.

You make a line of objects.

You partner makes a similar line - then adds one more.

Seems simple because it is simple. But it is also the beginnings of some very significant mathematics. 

- One to one correspondence (I make a line, you make a line) is the starting point for counting and giving a value to a collection of objects.

- "Adding one more" builds the idea of how numbers grow, how to build a sequence that increase by regular steps, how to think about addition.

- This is also pre-algebra: x, x+1....

- And we are learning about more than and less than.

Similar to Task 2 and 3, this task focuses on EYLF Outcome 2, "Children are connected with and contribute to their world."

One of the big ideas that is raised in this task is the idea of equality. In a mathematical sense, we are talking about the balance between two values. In a broader sense, we can take this conversation to include equality between people.

Equal shares is an important lesson in childhood. We can use this maths task to springboard into a discussion of how we should live with each other. From this, we can also develop strategies for dealing with inequality. 

Check out the miniMaths website:

http://www.minimaths.com.au

miniMATHS - 3. Same Same Different

This task - Same Same Different - is a fun game that children can play in many different contexts. It asks children to collect a set of three objects, two of which have a common feature that is absent in the third object. It is easy to see the potential for individual variation. And it also encourages children to think and to explain their thinking.

The suggested variations take the task into new places. "Same Same Same" asks the children to find common features in all three of their objects. "Different Different Different" turns the task upside down, requiring the children to identify differences between each of their objects.

And how is this task mathematical? Isn't it just a matching game? Well, at it's heart, this task is framing up some elementary concepts of algebra - finding common values, identifying sameness and difference, using logic and reasoning. These concepts are also important in numerical operations such as addition, subtraction, multiplication and division.

One aspect of the EYLF Outcome 2 looks at finding similarities and differences between people. Sound familiar? Isn't this the overt purpose of this task, finding same and different? This task can provide an ideal opportunity to promote conversations about diversity and individual differences. 

In the context of play, children can be encouraged to consider deep concepts and to start thinking about their own place in the world. Their connection with and contribution to the world is significant and should be nurtured through positive learning experiences such as this.

The miniMaths website can be found here:

http://www.minimaths.com.au 

miniMATHS - 2. Big Bigger Biggest

This is my favourite task. If I was in pre-k, I think I could have spent hours with sticks or rocks, lining them up in order of length. Actually, I probably did when I was.

The big idea behind this task is prompted by something I heard Nora Newcombe say at a conference last year. (Nora is a significant voice in mathematics education and research and has written heaps)  She said that her research had found that, while a sense of number was not something that babies are born with, they do seem to possess an innate sense of "magnitude" - something being "more than" something else.

So I was interested to include a task where students can develop this skill, looking at objects and arranging them based on an attribute such as length or area. This task also provides an opportunity to reverse the process, to look at small, smaller and smallest. 

It is not an accident that this task has used the nominative, comparative and superlative. This gives important links to grammar and language development in an informal context.

This task is linked to the second outcome from the Early Years Learning Framework:

Children are connected with and contribute to their world.

A big idea linked to this outcome is "change" - and this can be explored through the task by developing groups of objects that grow in a certain direction - by length, height, mass, area or in some other way. Children can see how they can add new objects to their sequence to make it grow. 

Once again, if anyone gives this one a go and has any feedback, please let me know. I would love to hear about it.

And here's the link to the website:

http://www.minimaths.com.au 

miniMATHS - 1. How many leaves?

from the "miniMATHS - Maths Inqiries in Nature" booklet

The tasks are organised based on the Early Years Learning Framework (EYLF). I linked each task to one of the five outcomes and put them in numerical order.

Outcome 1 - Children have a strong sense of identity

Task 1 - How many leaves?

The task is simple to set up. Draw a square in the dirt. See how many leaves you need to fill it up.

It is measuring area using informal units.

But you can take this to interesting places:

- move the leaves around in different arrangements to see if the number you need stays the same

- try using different types of leaves

- try using different sized shapes to fill up

- even try using the children themselves to fill up big spaces

One of the important ideas we want to experience is that "area is the space inside". And we can measure it.

from the "miniMATHS - Maths Inqiries in Nature" booklet

One of the important ideas presented in the EYLF outcome #1 relates to perspective. The "How many leaves?" task provides opportunities for students to explore the area of the square in many different ways and to understand that different people will find different solutions.

"Comparison" is another important concept that this task highlights, finding the area of a shape and comparing materials and strategies to complete it.

This task is ideal for small group interaction and is firmly based in the Nature Play pedagogy, using natural materials in a natural setting. Students can play with the idea and modify it to pursue their own questions and interests.

I would be interested in getting feedback from teachers who try out this task in their own schools. I would be particularly interested in hearing what the students have to say. 

Let me know.

Thanks.

Here's the miniMATHS website:

http://www.minimaths.com.au 

miniMATHS - Maths in Nature Inquiries

The Background

In 2018, my good friend Sam Hardwicke told me about the ACT Government Nature Play grants and suggested I should have a look at putting something in. I submitted an application on behalf of the Canberra Maths Association. We proposed to:

- write 10 inquiries/tasks/lessons/resources to be used outside in the natural environment
- run 10 workshops with preschools to share these resources

We were successful in our application and got the grant. A group of about 10 interested teachers from all levels of education, from early childhood, primary, secondary and tertiary, got together at the CMA conference in August and put together ideas for the 10 tasks. It was a productive time. I went away with pages of notes that I wrote up as the miniMATHS program.

The 10 tasks we came up with were:

1. How many leaves? - using informal units to measure area
2. Big, bigger, biggest - ordering by length
3. Same, same, different - identifying common features
4. One more - you make a number of objects, I'll make one more than you have
5. Shadows - moving objects to make new shadows
6. Stacking - building a pile of objects
7. Make a star - building a radial pattern
8. Does it float? - playing with floating and sinking
9. Rolling - looking at objects that roll
10. Repeat my pattern - if I make a pattern, can you extend it?

These 10 tasks were shaped into a book. I wanted some sort of printed product that teachers could physically carry with them into the bush, or at least outside into the playground. So some of the grant went into the printing of a book for every preschool in Canberra.

The 10 tasks have also been uploaded as a website that is available to anyone, not just ACT teachers:

http://www.minimaths.com.au 

Over the next week or so, I will post a bit more detail about each of the 10 tasks. Maybe you will find them useful. I would be keen to hear any feedback.

And any Canberra teachers - keep an eye out for the miniMATHS workshops rolling out over January and February.

Regards.