So that was 2014. All done.
Lots of interesting things happened here at AIM, including the Maths in Sport interviews way back in January and the Maths in Dance series in July. I might go back and read through a few of them, they were good.
I have decided not to do a similar series this January but will be taking a break and having a family holiday, so things will be pretty quiet here at AIM.
My plan for 2015 is to change the look of this blog a bit. I'm ready for a bit of a new look so stay tuned for how it will evolve.
I'm hoping to include more video and more student reflection - they are my two big targets for the coming year.
I will be teaching Year 2 in 2015 so there will be a noticeable shift towards that end of the teaching spectrum.
I also want to do some more of those interview series - perhaps looking at Maths in Music and Maths in Science.
Finally I am also hoping to engage with other classes out there and do some collaboration, particularly at the Year 2 level. I have one class in Melbourne lined up and ready to roll - the class of my (former) close friend and colleague Capitano Amazing (Richard Black) who has left the nation's capital and headed south. So if you are interested, get in contact.
My e-mail address is bruce.ferrington@radford.act.edu.au
Anyway, you all have a great holiday break and we will get back onto the blog sometime in late January.
Be good!
Sunday, 28 December 2014
Friday, 14 November 2014
End of Year Reports Wordle
As is my habit now, I have completed a Wordle using the text from my end of year reports.
Here's what my comments about my kids looks like:
I've posted it in "extra-large" size - I know it will go off the page a bit but it makes it easier to see.
I'm glad to see words like IDEAS and ABLE and GOOD coming across strongly. Good to know that these are the things I talk about most often in reports - especially the "ideas" thing.
Next level down we have INQUIRY and KNOWLEDGE and WORK and LEARNING and UNDERSTANDING and DISCUSSIONS. This is an interesting group - lots about the learning process. Glad to know that I am commenting about that.
A few maths words jump out - NUMBERS and MULTIPLICATION and DIGITS.
Something I do notice is that a lot of the Learner Profile words, that describe the individual and how they approach learning, and words that describe attitude are very small - something for me to reflect on. Next time I will...
Give it a go - drop your report comments into Wordle and see what comes up.
Here's what my comments about my kids looks like:
I've posted it in "extra-large" size - I know it will go off the page a bit but it makes it easier to see.
I'm glad to see words like IDEAS and ABLE and GOOD coming across strongly. Good to know that these are the things I talk about most often in reports - especially the "ideas" thing.
Next level down we have INQUIRY and KNOWLEDGE and WORK and LEARNING and UNDERSTANDING and DISCUSSIONS. This is an interesting group - lots about the learning process. Glad to know that I am commenting about that.
A few maths words jump out - NUMBERS and MULTIPLICATION and DIGITS.
Something I do notice is that a lot of the Learner Profile words, that describe the individual and how they approach learning, and words that describe attitude are very small - something for me to reflect on. Next time I will...
Give it a go - drop your report comments into Wordle and see what comes up.
Thursday, 13 November 2014
Fractions Workshop
This afternoon I attended a Canberra Maths Association (CMA - check out our website or Facebook page) workshop on Fractions being run by Caroline Evers.
Caroline is a Canberra teacher with a great breadth of experience from primary school up to college level. She had some great ideas that she shared. I want to show you one of them. It is beautiful in its simplicity but in the multiple layers of depth that you can uncover with it.
We chose some coloured A4 paper and had to fold it in half, then in quarters, then in eighths. I did mine like this:
We labelled the sections as we went along.
"This is a half."
"This is a quarter. I made it by getting half of a half."
"A half is bigger than a quarter."
"This is an eighth. It is half a quarter. It is a quarter of a half."
See this conversation? We are already laying the groundwork for multiplication and division of fractions!
The Caroline moved us on to thirds and sixths. Fold A4 paper in half, then into thirds. Label as you go:
Ah-ha! So, one third of a half is a sixth!
You guessed it - a fifth of a half is a tenth. And there are 5 tenths in a half. Sounds like equivalent fractions to me.
And it is all so visual.
You could do this with early years classes to show what fractions look like and get that understanding of what a fraction of a whole is.
You could use it with upper primary classes to look at equivalent fractions, adding and subtracting fractions and even looking at comparing areas of rectangles (e.g. - all the different ways you make an eighth of a piece of A4 will have the same area).
And you can use it in high school when you start to multiply and divide fractions with different denominators. Or with fractions in algebra. Or with...
Ah! It looks so simple on the outside but this activity has lots of possibilities. Give it a go and see what you can do with it...
Caroline is a Canberra teacher with a great breadth of experience from primary school up to college level. She had some great ideas that she shared. I want to show you one of them. It is beautiful in its simplicity but in the multiple layers of depth that you can uncover with it.
We chose some coloured A4 paper and had to fold it in half, then in quarters, then in eighths. I did mine like this:
We labelled the sections as we went along.
"This is a half."
"This is a quarter. I made it by getting half of a half."
"A half is bigger than a quarter."
"This is an eighth. It is half a quarter. It is a quarter of a half."
See this conversation? We are already laying the groundwork for multiplication and division of fractions!
1/2 x 1/4 = 1/8
But we could also compare and combine the different fractions to see which were biggest, smallest, equal etc.
The Caroline moved us on to thirds and sixths. Fold A4 paper in half, then into thirds. Label as you go:
Ah-ha! So, one third of a half is a sixth!
1/2 ÷ 3 = 1/6
And then we did fifths and tenths:
You guessed it - a fifth of a half is a tenth. And there are 5 tenths in a half. Sounds like equivalent fractions to me.
And it is all so visual.
You could do this with early years classes to show what fractions look like and get that understanding of what a fraction of a whole is.
You could use it with upper primary classes to look at equivalent fractions, adding and subtracting fractions and even looking at comparing areas of rectangles (e.g. - all the different ways you make an eighth of a piece of A4 will have the same area).
And you can use it in high school when you start to multiply and divide fractions with different denominators. Or with fractions in algebra. Or with...
Ah! It looks so simple on the outside but this activity has lots of possibilities. Give it a go and see what you can do with it...
Monday, 10 November 2014
Playing with Probability
Having started the conversation about probability, I was keen to push on and get our hands dirty with a bit of investigation.
First thing we looked at was rolling dice. Each number has an equal chance of being rolled. How would this play out with 100 rolls? And then if we added the 100 rolls from 24 students?
So we got to work. Would the numbers come out evenly?
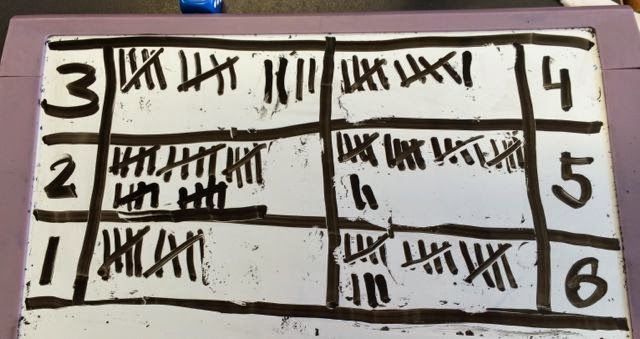
Here's some data recording:
And once we had data, we needed to display it as a graph so that we could see our data clearly:
So we then combined all our data and put together the results on Excel.
You will notice that 24 students rolling a dice 100 times each gave us a total number of dice rolls of .... 2419?
Much discussion followed about why we got these results. Why weren't they all even? Why was "3" so low? Why was "2" so high? How could we do it differently?
Next we made some spinners. This was a bit of fun. Simple to make as well.
I printed off some hexagon shapes which we stuck onto cardboard, coloured in different ways (one spinner unequal chances, one spinner equal chances, one free choice) then stuck through a tooth pick.
First thing we looked at was rolling dice. Each number has an equal chance of being rolled. How would this play out with 100 rolls? And then if we added the 100 rolls from 24 students?
So we got to work. Would the numbers come out evenly?
Here's some data recording:
This one looked a bit random - the numbers are in a strange order -
but it works.
A bit untidy but you get the idea.
So, did you record 100 rolls? Let's see if that adds up...
Don't you just love girls? They are soooo organised!
And once we had data, we needed to display it as a graph so that we could see our data clearly:
Yep - beautiful presentation, accuracy, colour, neatness...
So we then combined all our data and put together the results on Excel.
You will notice that 24 students rolling a dice 100 times each gave us a total number of dice rolls of .... 2419?
Much discussion followed about why we got these results. Why weren't they all even? Why was "3" so low? Why was "2" so high? How could we do it differently?
Next we made some spinners. This was a bit of fun. Simple to make as well.
I printed off some hexagon shapes which we stuck onto cardboard, coloured in different ways (one spinner unequal chances, one spinner equal chances, one free choice) then stuck through a tooth pick.
You will notice that 24 students rolling a dice 100 times each gave us a
total number of dice rolls of .... 2419?
Much discussion followed about why we got these results. Why weren't
they all even? Why was "3" so low? Why was "2" so high? How
could we do it differently?
Next we made some spinners. This was a bit of fun. Simple to make as
well.
I printed off some hexagon shapes which we stuck onto cardboard,
coloured in different ways (one spinner unequal chances, one spinner equal
chances, one free choice) then stuck through a tooth pick.
Here's the template we used
Here's what mine looked
like
The kids were so much more creative than me...
And then we had to invented some games of chance. Here's a few
action shots of games in progress:
And a lot of fun was had by all.
It's simple. It's easy. It relates to the Australian Curriculum:
List outcomes of chance
experiments involving
equally likely outcomes and
represent probabilities
of those outcomes using
fractions (ACMSP116)
All good.
Friday, 7 November 2014
Simple Probability
We've started doing some probability work this week - (hmmm... just "happen" to do probability to coincide with Melbourne Cup week - what are the chances of that?).
As ever, we spent some time looking at the language of probability. I was keen to hear what the kids would say about the words we use to describe chance.
We started with a continuum from 0-1 and placed a few words on the line where we thought they would go.
It looked a bit like this.
And some close ups:
Then we talked about the meanings of the words and how they related to chance.
Here's a few of the comments the kids made:
As ever, we spent some time looking at the language of probability. I was keen to hear what the kids would say about the words we use to describe chance.
We started with a continuum from 0-1 and placed a few words on the line where we thought they would go.
It looked a bit like this.
And some close ups:
Then we talked about the meanings of the words and how they related to chance.
Here's a few of the comments the kids made:
It is impossible, certain and 50-50 or half.
When there’s an even amount of choices and an even amount of things in that choice then
it’s impossible to predict which one it will be.
There’s a lot of words for 50-50.
Halfway between impossible and certain there’s
an even chance of it not happening or happening.
There’s always chance in whatever you do.
If you’re kicking a footy there’s a chance that it will go to whoever you’re
kicking it to or not.
There are different words for impossible,
certain and half because they’re also things in between those.
In probability there are fractions
involved. You can’t go over the 1 point.
Buckley’s means “no chance”.
Almost everything we do in life has a bit
of chance.
I don’t think there are things that are
certain or things that are impossible.
So, language is always a good place to start. We were able to assess prior knowledge, explore a few new ideas a little bit and generally get the ball rolling. It also gave us some direction for where we would go next.
So, language is always a good place to start. We were able to assess prior knowledge, explore a few new ideas a little bit and generally get the ball rolling. It also gave us some direction for where we would go next.
Subscribe to:
Posts (Atom)